Wheel factorization
Wheel factorization is a graphical method for manually performing a preliminary to the Sieve of Eratosthenes that separates prime numbers from composites. Start by writing the natural numbers around circles as shown below. Prime numbers in the innermost circle have their multiples in similar positions as themselves in the other circles, forming spokes of primes and their multiples. Multiples of the prime numbers in the innermost circle form spokes of composite numbers in the outer circles.
Contents |
Procedure
- Find the first few prime numbers. They are known or can be found quickly using Sieve of Eratosthenes.
- Multiply the prime numbers together to give the result n.
- Write 1 to n in a circle. This will be the inner-most circle.
- Taking x to be the number of circles written so far, continue to write xn + 1 to xn + n in another circle around the inner-most circle, such that xn + 1 is in the same position as (x − 1)n + 1.
- Repeat step 4 until the largest number to be tested for primality.
- Strike off the number 1.
- Strike off the spokes of prime numbers (found in step 1) with its multiples without striking off the numbers in the inner-most circles.
- Strike off the spokes of all multiples of prime numbers found in step 1.
- The remaining numbers in the wheel contain mostly prime numbers. Use other methods such as Sieve of Eratosthenes to remove the remaining non-primes.
Example
1. Find the first 2 prime numbers—2 and 3.
2. n = 2 · 3 = 6
3.
1 2 3 4 5 6
4. x = 1. xn + 1 = 1 · 6 + 1 = 7. (x + 1)n = (1 + 1) · 6 = 12. Write 7 to 12 with 7 aligned with 1.
1 2 3 4 5 6 7 8 9 10 11 12
5. x = 2. xn + 1 = 2 · 6 + 1 = 13. (x + 1)n = (2 + 1) · 6 = 18. Write 13 to 18. Repeat for the next few lines.
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30
6 and 7. Sieving
12 3 4 5 6 78910 11 12 13141516 17 18 19202122 23 24 25262728 29 30
8. Sieving
12 3456789101112131415161718192021222324252627282930
9. Resultant list contains a non-prime 25. Use other methods of sieve to arrive at
2 3 5 7 11 13 17 19 23 29
Analysis and Computer implementation
Given a number k > n, we know that it is not prime if k mod n and n are not relatively prime. The fraction of numbers that the wheel sieve eliminates is 1 - phi (n) / n, which is also the efficiency of the sieve. From the properties of phi, it can easily be seen that the most efficient sieve smaller than x is the one where 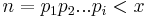 and
and 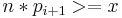 . It can also be demonstrated that this efficiency rises very slowly for large n.
. It can also be demonstrated that this efficiency rises very slowly for large n.
To be of maximum use on a computer, we want the numbers that are smaller than n and relatively prime to it as a set. Using a few observations, the set can easily be generated :
- Start with
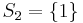 , which is the set for
, which is the set for 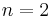 .
. - Let
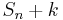 be the set where k has been added to each element of
be the set where k has been added to each element of 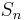 .
. - Then
![S_{n p_{i%2B1}} = F_{p_{i%2B1}} [S_n \cup S_n %2B n \cup S_n %2B 2n \cup ... \cup S_n %2B n (p_{i%2B1} - 1)]](/2012-wikipedia_en_all_nopic_01_2012/I/fc0ddc2b643dd78b7c51b1e9bf0bad56.png) where
where 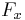 represents the operation of removing all multiples of x.
represents the operation of removing all multiples of x. - 1 and
 will be the two smallest of
will be the two smallest of 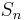 when
when 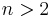 removing the need to compute prime numbers separately
removing the need to compute prime numbers separately - The set is symmetrical around
 , reducing storage requirements.
, reducing storage requirements.
External links
- Wheel Factorization
- Improved Incremental Prime Number Sieves by Paul Pritchard
|
|||||||||||||||||||||||||||||